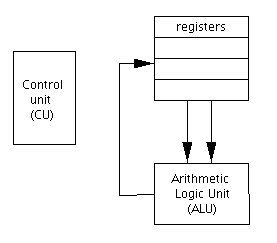
CPU Subunits - Note the two buses connecting the registers to the ALU.
This is due to the fact that ALU operations like addition operate on two data values,
whereas the result is a single value (the bus from the ALU back to the registers)
In order to better understand this cycle, we will investigate a very simple simulation of a CPU datapath. The simulator has 4 registers and an ALU (with operations for addition, subtraction, bitwise-AND, and bitwise-OR). This simplified CPU does not have a Control Unit. Instead, we will act as the control unit, selecting the input registers, the ALU function, and the output register for the result, by clicking on the appropriate knobs in the simulator.
To start, open a second browser window and in this window go to the simulator at this site -- http://www.prenhall.com/reed/Chapter14/datapath.html
You will see a page with the simulator as shown.
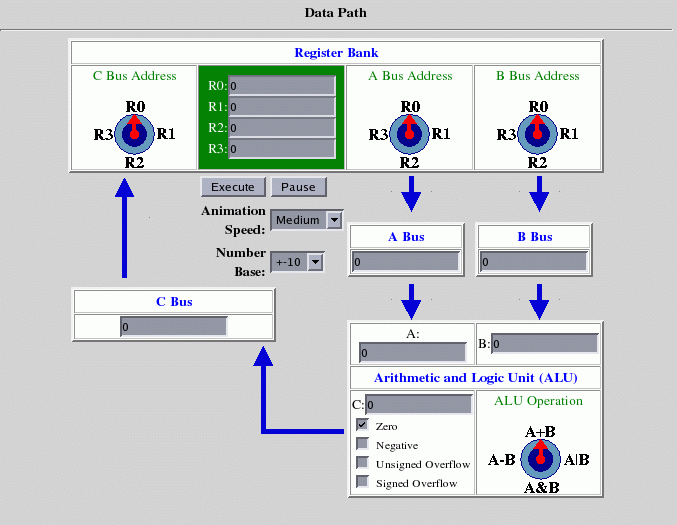
The ALU will do four basic operation as described above. We select which operation it will do by the use of the knob labeled "ALU Operation."
Control through this datapath is started by clicking the "Execute" button.
As an example, set registers 0 and 1 to be 43 and -296, respectively. (We are following the example on page 253 of the text.) Then, set the A bus knob to point to R0, and the B bus knob to R1. Set the C bus knob to R2. Leave the ALU knob in its default position, i.e. at the "A+B" position. Then, click the "Execute" button. The simulator will slowly show how data travels from the registers along the buses to the ALU and then how the result travels along bus C back to the registers. Closely watch how this datapath is executed before moving on, so that you understand what the simulator is showing.